Sign Conventions for Magnetic Fields

The MAD program uses the following Taylor expansion for the normal and skewed field components respectively in the mid-plane $y$=0, described in SLAC-75:

$$B_y(x,0)=\sum_{k=0}^{\infty}\frac{B_{kn}x^k}{k!}, \qquad B_x(x,0)=\sum_{k=0}^{\infty}\frac{B_{ks}x^k}{k!}.$$

Note the factorial in the denominator. The field coefficients have the following meaning:

$B_{0n}$

Normal dipole field component. The component is positive, if the field points in positive $y$ direction. A positive field bends a positively charged particle travelling in positive $s$-direction to the right.

$B_{0s}$

Skew dipole field component. The component is positive, if the field points in negative $y$ direction. A positive field bends a positively charged particle travelling in positive $s$-direction down.

$B_{1n}$

Normal quadrupole field component $B_{1n}=\partial B_y/\partial x$. The component is positive, if $B_y$ is positive on the positive $x$-axis. A positive value corresponds to horizontal focussing of a positively charged particle.

$B_{1s}$

Skew quadrupole field component $B_{1s}=\partial B_x/\partial x$. The component is positive, if $B_x$ is negative on the positive $x$-axis.

$B_{2n}$

Normal sextupole field component $B_{2n}=\partial^2 B_y/\partial x^2$. The component is positive, if $B_y$ is positive on the $x$-axis.

$B_{2s}$

Skew sextupole field component $B_{2s}=\partial^2 B_x/\partial x^2$. The component is negative, if $B_x$ is positive on the $x$-axis.

$B_{3n}$

Normal octupole field component $B_{3n}=\partial^3 B_y/\partial x^3$. The component is positive, if $B_y$ is positive on the positive $x$-axis.

$B_{3s}$

Skew octupole field component $B_{3s}=\partial^3 B_x/\partial x^3$. The component is negative, if $B_x$ is positive on the $x$-axis.

All derivatives are taken on the $x$-axis. Using this expansion and the curvature $h$ of the reference orbit, the longitudinal component of the vector potential for a magnet with mid-plane symmetry is to order 4:

$$\begin{array}{rclcl} A_s=&+&B_{0n}\left(x-\frac{hx^2}{2(1+h... ...1}{24}(x^{4}-6x^2y^2+y^{4})+\ldots\right) + \ldots \end{array}$$

Taking $\mathrm{curl} A$ in curvilinear coordinates, the field components can be computed as

$$\begin{array}{rclcl} B_x(x,y)= &+&B_{1n}\left(y+\frac{h^2}... ...eft(\frac{1}{6}(x^{3}-3xy^2)+\ldots\right) + \ldots \end{array}$$

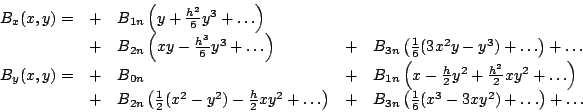
One can easily verify that both $\mathrm{curl} B$ and $\mathrm{div} B$ are zero to the order of the $B_3$ term. Introducing the magnetic rigidity $B \rho$, the multipole coefficients are computed as

$$K_{kn}=eB_{kn}/p_0=B_{kn}/B\rho,\qquad K_{ks}=eB_{ks}/p_0=B_{ks}/B\rho.$$

Note that the $K_k$ have the same sign as the corresponding field components $B_k$. The signs will be changed due to the sign of particle charges and the direction of travel of the beam.